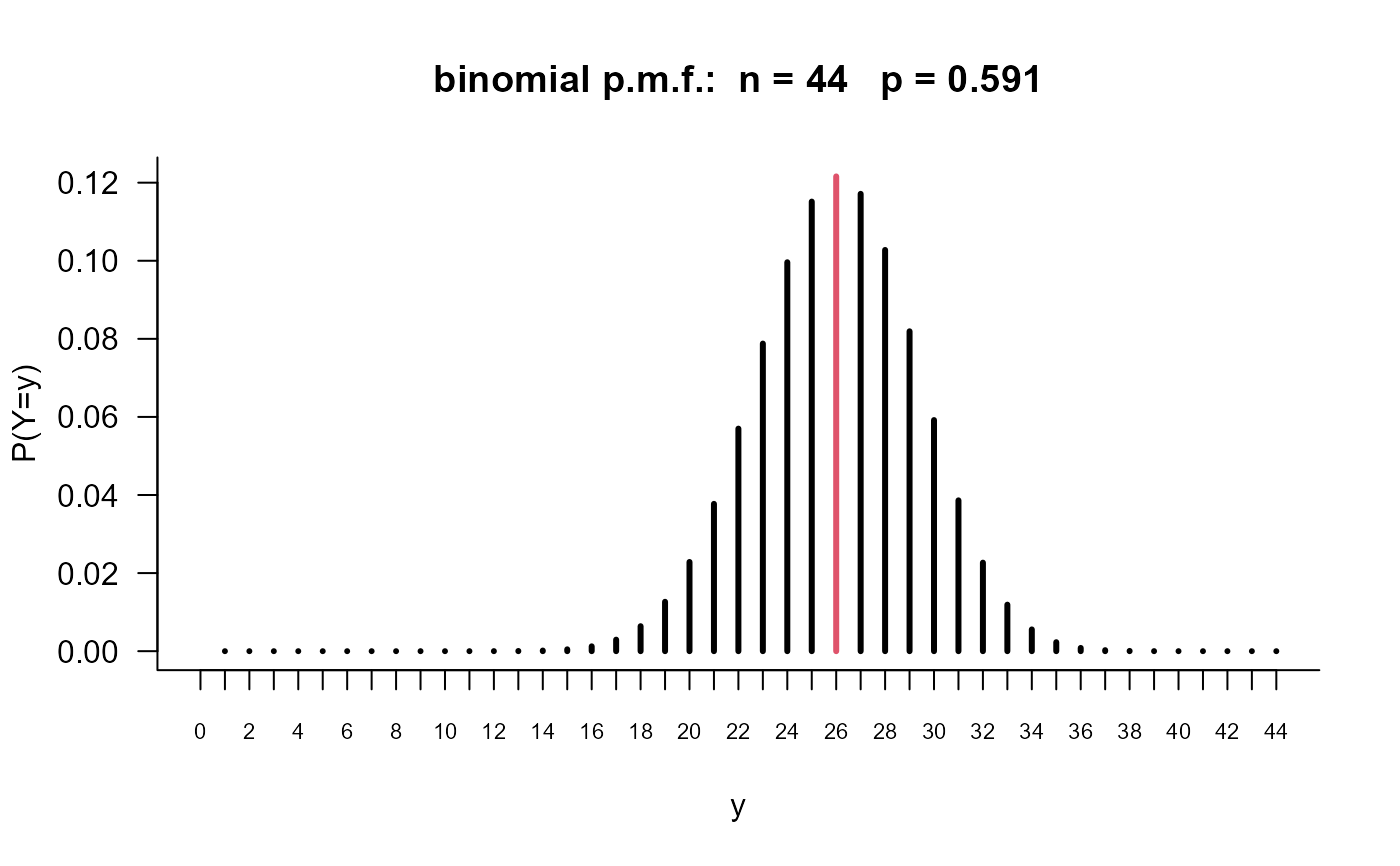Binomial p.m.f. movie

A movie to illustrate how the probability mass function (p.m.f.) of a binomial (n, p) random variable depends on n and p. For more discrete distributions, including the geometric and Poisson distributions, use smovie::movies() and click on the Discrete menu. This is based on the discrete function. (If you have not installed the smovie package then use install.packages("smovie") to install it.)

Usage

binomial_pmf_movie(
  starting_n = 1,
  starting_p = 1/2,
  delta_n = 1,
  delta_p = 0.05,
  observed_value = NA
)

Arguments

starting_n

A numeric scalar. The value of n for the first graph.

starting_p

A numeric scalar. The value of p for the first graph.

delta_n

A numeric scalar. The amount by which n is increased (or decreased) after one click of the + (or -) button in the parameter window.

delta_p

A numeric scalar. The amount by which p is increased (or decreased) after one click of the + (or -) button in the parameter window.

observed_value

A non-negative integer. If observed_value is supplied then the corresponding line in the plot of the p.m.f. is coloured in red. If observed_value is not an integer then round(observed_value) is used.

Value

Nothing is returned, only the animation is produced.

Details

The probability mass function of a binomial random variable with parameters \(n\) (the number of Bernoulli trials performed) and \(p\) (the probabilities of success on a each trial) is plotted. The values of \(n\) and \(p\) can be changed by clicking on the relevant buttons.

See also

stat0002movies: general information about the movies.

Examples

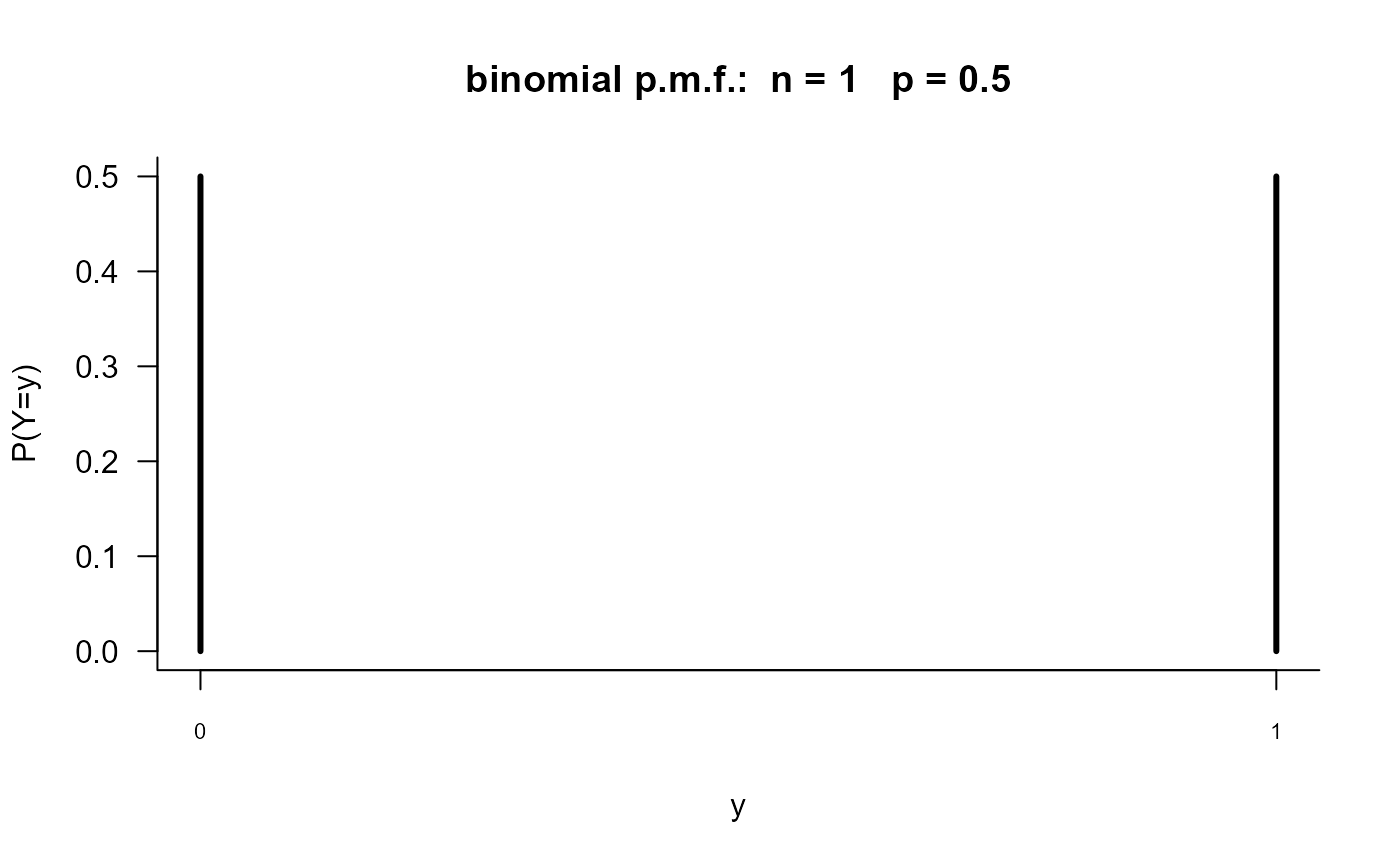
binomial_pmf_movie()


# Increase n and see what happens
binomial_pmf_movie(delta_n = 10)

# Sample size of the Aussie births data (26 boys, 18 girls)
binomial_pmf_movie(starting_n = 44, starting_p = 0.1, delta_p = 0.1,
                   observed_value = 26)


# Start at p = 0.591 (approximately 26/44)
binomial_pmf_movie(starting_n = 44, starting_p = 0.591, delta_p = 0.01,
                   observed_value = 26)